Problem
https://leetcode.com/problems/maximum-depth-of-binary-tree/
Maximum Depth of Binary Tree - LeetCode
Can you solve this real interview question? Maximum Depth of Binary Tree - Given the root of a binary tree, return its maximum depth. A binary tree's maximum depth is the number of nodes along the longest path from the root node down to the farthest leaf
leetcode.com
Hint
Try using recursive approach.
Solution
To solve this problem, we can utilize a recursive approach. Let's outline the steps:
- If the given binary tree is empty (i.e., the root is null), the depth is zero. We have reached the base case, so we return 0.
- Otherwise, we calculate the maximum depth of the left and right subtrees recursively. We make two recursive calls, one for the left child and one for the right child.
- We compare the depths of the left and right subtrees and return the maximum depth between them plus one. The "+1" accounts for the current node in the path.
Code
Simple
class Solution {
public:
int maxDepth(TreeNode* root) {
if (root == nullptr) return 0;
int leftMax = maxDepth(root->left);
int rightMax = maxDepth(root->right);
return max(leftMax, rightMax) + 1;
}
};
Whole Code with Test Case
// https://leetcode.com/problems/maximum-depth-of-binary-tree/
#include <iostream>
#include <algorithm>
using namespace std;
// Definition for a binary tree node.
struct TreeNode {
int val;
TreeNode *left;
TreeNode *right;
TreeNode() : val(0), left(nullptr), right(nullptr) {}
TreeNode(int x) : val(x), left(nullptr), right(nullptr) {}
TreeNode(int x, TreeNode *left, TreeNode *right) : val(x), left(left), right(right) {}
};
class Solution {
public:
int maxDepth(TreeNode* root) {
if (root == nullptr) return 0;
int leftMax = maxDepth(root->left);
int rightMax = maxDepth(root->right);
return max(leftMax, rightMax) + 1;
}
};
int main() {
Solution s = Solution();
TreeNode node15(15);
TreeNode node7(7);
TreeNode node20(20, &node15, &node7);
TreeNode node9(9);
TreeNode root(3, &node9, &node20);
cout << s.maxDepth(&root) << endl;
}
Complexity
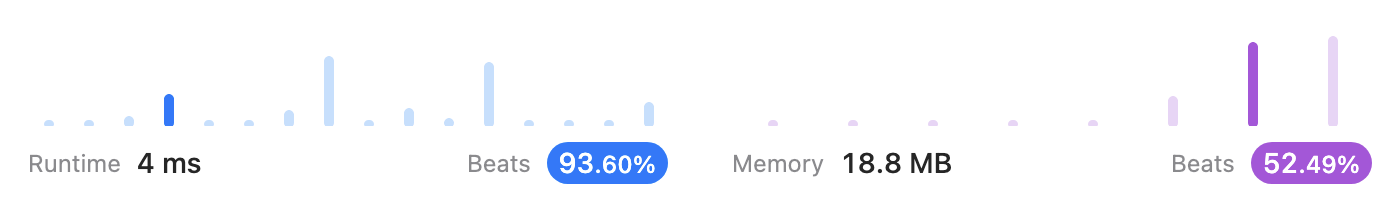
Time
Time complexity will be O(n).
Space
Space complexity will be O(1).
Best Solution (Pinned)
class Solution {
private:
int dfs(TreeNode* root, int level) {
if (root == 0) return level;
int Max = level;
Max = max(Max, dfs(root -> left, level + 1));
Max = max(Max, dfs(root -> right, level + 1));
root -> left = 0;
root -> right = 0;
return Max;
}
public:
int maxDepth(TreeNode* root) {
if (!root) return 0;
return dfs(root, 0);
}
};
Overall